Every religion has some people claiming that their religion is the only path. Some Christians say that only through Jesus one can reach God. Some Muslims state that if you are not Muslim you will not enter heaven. Some Jews say they're the only chosen ones. Some atheists claim they're the only ones with brains. Some Hindus believe they're the only ones who have the right to perform religious ceremonies on the basis of their birth, etc. This has unfortunately led to religious intolerance and turned many people away from even considering topics related to spirituality and has thus deprived them of a richer, fuller and more balanced experience of life. In trying to create bridges by which we can approach one another and appreciate each other's faiths, we can maybe draw some analogies and learn some lessons from non-Euclidean geometries.
Euclidean geometry is the familiar geometry we all learned in secondary school. It was first formalized by the Alexandrian Greek Euclid around 300 B.C. starting from a small set of basic, intuitively evident statements (postulates). Most of our day-to-day world is based upon it. It is the geometry of the engineers, designers, architects and some physicists. However, in the late 19th century, a few European mathematicians established new self-consistent systems of geometry that led to different conclusions and properties by changing Euclid's postulates. Among the most famous ones are the hyperbolic and the elliptic geometries.
To get an idea of how these geometries differ from the usual Euclidean geometry, you can think of Euclidean geometry as what you would expect if you worked geometry on a flat piece of paper. Elliptic geometry is what you would get if you worked geometry on a sphere. Change that sphere into a saddle and you are now working on hyperbolic geometry.
As mentioned previously, the conclusions you arrive by working in these different types of geometries are not always the same. One typical example of this is the sum of the internal angles of a triangle. If you measure the three angles inside a triangle in the Euclidean geometry, as we all learned back in school, the sum should always be 180 degrees. Do this on the surface of a sphere (elliptic geometry) and you'll end up with a sum greater than 180 degrees. On a saddle (hyperbolic geometry), the sum will be smaller. See figure 1.
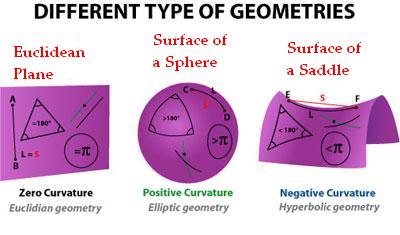
Figure 1 (Source)
Now, up to the 19th century, to consider that there could be other types of geometries beside that of Euclid was almost heretic. Does this begin to sound like religion? In fact, the great German mathematician Carl Friedrich Gauss never published the work he developed in non-Euclidean geometry out of fear of losing his prestige, and it was one of his students, Georg Bernhard Riemann, who actually got the credit later on.
Imagine now that you have three friends: one who has been exposed all his life only to Euclidean geometry, one to the elliptic and one to the hyperbolic. One will defend with his life that the sum of the internal angles of a triangle is always greater than 180 degrees, while the other will challenge that it is always less, and the other one still will assert that it is always equal to 180 degrees. All will quote from their trusted text books and teachers, and by line of reasoning, will try to prove to each other that they're right and the other one is wrong. All of them are right to a certain extent, even though logic would dictate that a simple sum cannot be simultaneously more than, equal to, and smaller than a certain quantity. But unless they have a deep knowledge of their geometries and an understanding of higher mathematics to see the underlying manifold (a flat surface, a sphere or a saddle) on which they're doing their mathematics and the universal principles that actually apply to all of them to thus see why all their conclusions may be right; they will either fight eternally or, in a desperate attempt to find peace and unity, wrongly conclude that anything anybody says about geometry is correct.
Similarly, unless we develop a deep realization of our own spiritual paths to see the underlying universal spiritual principles that apply to all of us, beyond the external differences of form in ritual, we will either develop egoistic, sectarian views that will only lead to hatred and hence take us further away from the goal we're really trying to reach, or in a desperate attempt to agree with each other, we will become whimsical and water down the process of spiritual advancement leading us nowhere. It is important that we recognize how and why God has come in different places at different times to deliver the same spiritual messages; how He, as any intelligent speaker would, adapts these messages according to the time, place and circumstance of the audience.
Throughout the different types of faith, emphasis is always given to developing love for God, compassion toward all living entities and chanting the names of God. To understand the details to perform this properly, it is recommended one approach a genuine and spiritually realized teacher in a mood of earnest and humble inquiry and eager to serve the instructions given by him/her.
How certain seemingly contradictory statements between religions remain true is an interesting topic of research that cannot be dealt with by mere academic study, but only by personal realization of the spirit of the instructions. Truly advanced people in the science of spirituality (those who know the higher mathematics of non-Euclidean geometries) can actually have meaningful interfaith exchanges, for they are able to appreciate the mercy of God in its multifarious forms and the different expressions of devotion from people all over the world.